Definition of a 6s Process

The term 6s (Six Sigma) originates from statistical modeling of manufacturing processes. The maturity of a manufacturing process can be described by a sigma rating indicating its yield or the percentage of defect-free products it creates—specifically, to within how many standard deviations of a normal distribution the fraction of defect-free outcomes corresponds. A 6s process implies that six standard deviations fit between the mean and the nearest specification limit.

Specifically, say that there are six standard deviations—represented by the Greek letter σ (sigma)—between the mean—represented by μ (mu)—and the nearest specification limit. As process standard deviation goes up, or the mean of the process moves away from the center of the tolerance, fewer standard deviations will fit between the mean and the nearest specification limit, decreasing the sigma number and increasing the likelihood of items outside specification. According to a calculation method employed in process capability studies, this means that practically no items will fail to meet specifications.

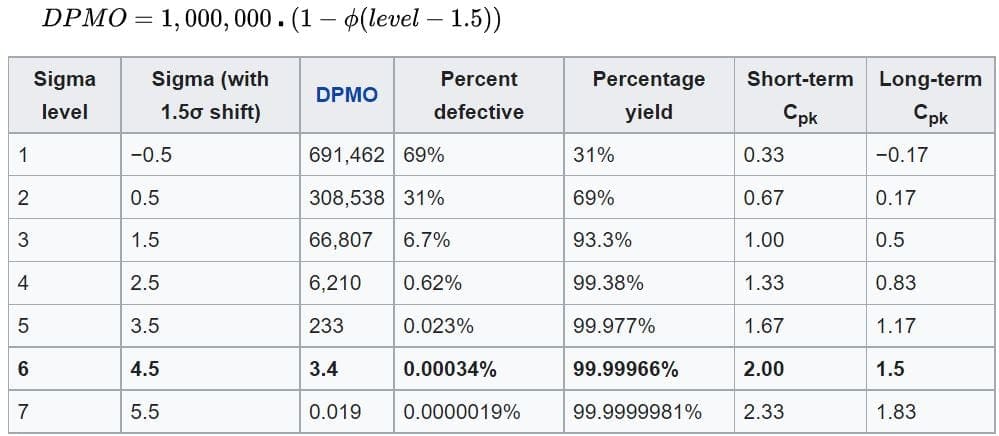
The table below gives long-term DPMO (Defects Per Million Opportunities) values corresponding to various short-term sigma levels. These figures assume that the process mean will shift by 1.5 sigma toward the side with the critical specification limit. In other words, they assume that after the initial study determining the short-term sigma level, the long-term Cpk value will turn out to be 0.5 less than the short-term Cpk value. So, now for example, the DPMO figure given for 1 sigma assumes that the long-term process mean will be 0.5 sigma beyond the specification limit (Cpk = –0.17), rather than 1 sigma within it, as it was in the short-term study (Cpk = 0.33). Note that the defect percentages indicate only defects exceeding the specification limit to which the process mean is nearest. Defects beyond the far specification limit are not included in the percentages.

The formula used here to calculate the DPMO is thus

6s process in terms of DPMO

References

Wikipedia. Six Sigma. https://en.wikipedia.org/wiki/Six_Sigma#Tools_and_methods