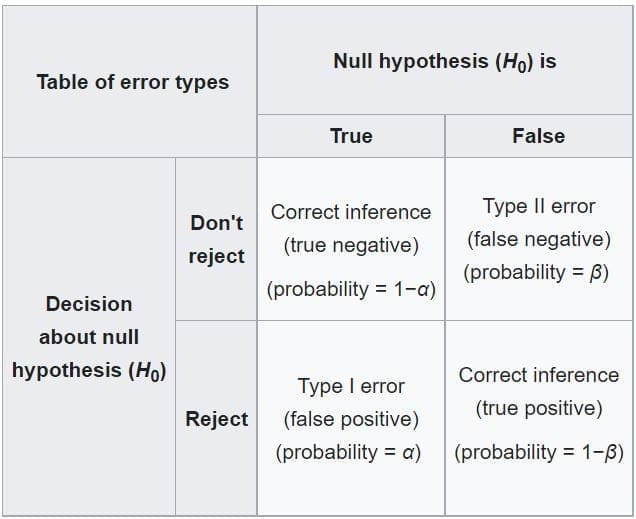
In statistical hypothesis testing, a type l error is the rejection of a true null hypothesis (also known as a “false positive” finding or conclusion), while a type II error is the non-rejection of a false null hypothesis (also known as a “false negative” finding or conclusion). A type I error is finding an imagined difference where none actually exists or it can be defined as the rejection of a true null hypothesis as the result of a test procedure.
Intuitively, Type l errors can be thought of as errors of commission, For example; a researcher unluckily concludes that something is the fact. Consider a study where researchers compare a drug with a placebo. If the patients who are given the drug get better than the patients given the placebo by chance, it may appear that the drug is effective, but in fact the conclusion is incorrect. In reverse, type II errors are errors of omission. In the example above, if the patients who got the drug did not get better at a higher rate than the ones who got the placebo, but this was a random fluke, that would be a type II error. The consequence of this type of error depends on the size and direction of the missed determination and the circumstances. An expensive cure for one in a million patients may be inconsequential even if it truly is a cure.
Example
Since in a real experiment it is impossible to avoid all type I and type II errors, it is important to consider the amount of risk one is willing to take to falsely reject H0 or accept H0. The solution to this question would be to report the p-value or significance level α of the statistic. For example, if the p-value of a test statistic result is estimated at 0.0596, then there is a probability of 5.96% that we falsely reject H0. Or, if we say, the statistic is performed at level α, like 0.05, then we allow to falsely reject H0 at 5%. A significance level α of 0.05 is relatively common, but there is no general rule that fits all scenarios.
References
Wikipedia. https://en.wikipedia.org/wiki/Type_I_and_type_II_errors